I thought I’d circle back to discuss the topic of compounders and return on capital. I wrote a few posts about earlier this year, and there have been numerous comments and questions.
In this post, I want to discuss the actual math behind the compounders, to try and show why return on capital is so important to long term business owners (which is what we are as stockholders).
To recap what I mentioned earlier, I usually put investments in two broad categories, but ideally, I’m looking for:
- A business that can produce high returns on capital
- A business that can reinvest a large portion of earnings at similar high rates
- A business run by good management, who will allocate the excess cash in a value creating way (preferably management owns a large stake in the business themselves, aligning interests)
These three factors combine to create the rate that the business compounds intrinsic value over time.
The Math Behind Compounding Intrinsic Value
The math is simple.
To briefly review, I find it helpful to use a back of the envelope formula as a way to think about the rate at which a business is compounding its intrinsic value. Basically, a business will grow its intrinsic value at a rate that equals the product of two factors: the incremental return on invested capital (ROIC) and the reinvestment rate.
A simple example: a business that can reinvest 50% of its earnings back into the business at a 12% return on investment will compound the intrinsic value of the enterprise at 6% annually (50% x 12%). See this post for more discussion on this.
Let’s look at a hypothetical example of 2 businesses (Company A and Company B):
- Company A produces 20% ROIC and can reinvest 100% of its earnings
- Company B produces 20% ROIC and can reinvest 50% of its earnings
In both examples, we’ll assume the investment is a long term investment over 15 years. Many investments don’t last this long, but in this exercise we are imagining ourselves as a long term partner in the business, and this is how I happen to think about stocks anyhow. And business owners don’t trade in and out of businesses every year or two. We’ll look at various starting and ending valuations, and we’ll see how both the valuation (the P/E ratios) as well as the quality (ROIC) impact our investment returns over the 15 years.
Example 1—Company A
In this example, we’ll assume earnings are cash earnings to make things simple. Let’s assume the business produces $1.00 of earnings per share, and let’s say Company A has a stock price of $25.00 (a P/E of 25). Let’s assume the business is a growing enterprise and it can reinvest 100% of its earnings back into the business at a rate of 20% after tax (20% Return on Capital). The business sells a niche product to a growing market of customers that rely on Company A exclusively, thus the business has a nice competitive advantage over potential competitors. This “moat” allows them to achieve 20% returns on capital for the next 15 years.
Let’s take a look at the value of the business in 15 years:
- Year 1 EPS = $1.00
- Year 15 EPS = $15.40
Pretty simple… if the business has an ROIC of 20% and can reinvest 100% of their earnings, then earnings will grow at 20% over time, and the growth of the intrinsic value of the business will also approximate this 20% annual growth rate.
Now, let’s say we paid 25 times earnings for this business 15 years ago ($25 per share). Let’s take a look at what the value of our stock (and the 15 year CAGR) will be at various P/E multiples (remember we paid $25 per share 15 years ago for Company A):
- 10 P/E: $154.00 per share (12.9% CAGR)
- 15 P/E: $231.00 per share (16.0% CAGR)
- 20 P/E: $308.00 per share (18.2% CAGR)
So for this wonderful business, even paying 25 times earnings worked out to a stellar return for shareholders of around 13% annually for 15 years even as the P/E multiple contracted from 25 all the way down to 10, which would be a very low multiple for a great business like this.
Example 2—Company B
Let’s assume that this business–Company B–reinvests half of its earnings at a rate of return (ROIC) of 20% and pays out the other 50% as a dividend. In this case, the intrinsic value of the enterprise will compound at 10% annually (20% ROIC times the 50% reinvestment rate equals a 10% growth rate). Let’s assume we paid the same price as the first business (25 times earnings). Let’s assume the same $1 starting EPS.
In 15 years, EPS will equal $4.17 per share (assuming a constant 20% return on incremental capital and a 50% reinvestment rate). Let’s also assume a constant 2% dividend yield, since Company B can pay out a portion of earnings as dividends, unlike Company A which reinvested all of its earnings.
- 10 P/E: $41.77 (5.5% CAGR)
- 15 P/E: $62.66 (8.3% CAGR)
- 20 P/E: $83.54 (10.4% CAGR)
So both of the above businesses produce 20% returns on invested capital, but Company A is clearly the superior investment if both are priced around the same level. This is simply because company A has twice the level of investment opportunity, as it can reinvest all of its earnings at 20%, whereas company B can only invest half of its earnings at 20%.
Logically, this makes perfect sense. If two businesses (Company A and Company B) have opportunities to make 20% returns on incremental investments, but Company A can invest twice as much as Company B at that 20% rate of return, then company A will create much more value over time for its owners than Company B.
Both companies above will show up in screeners as businesses that produce 20% ROIC, but one is clearly superior to the other because it can retain and reinvest a higher portion of its earnings, and thus will compound intrinsic value much faster.
Picking the “Right Business” is More Important than Picking the “Right Multiple”
In Part 3 of this series, I mentioned that ROIC is far and away the most important factor to consider, as the math (the back of the envelope formula) shows. A business that produces 6% ROIC will not compound intrinsic value regardless of how much they can invest back into the business (in fact, a business that produces low returns would be better off not reinvesting, as owners could likely reallocate those earnings at higher rates elsewhere).
So a business that can produce above average returns on capital is crucial when it comes to compounding value.
But I also wanted to demonstrate that picking the right business (i.e. the one that can invest large amounts of capital at attractive rates of return) is far more important than paying the lowest multiple to current earnings.
Notice in the simple example above, I assumed that we paid a P/E multiple of 25 for both businesses. Obviously, at the same price to earnings ratio, the business that can compound intrinsic value faster will create better returns for shareholders. But let’s compare investment results if we pay a lot less for Company B (the inferior compounder).
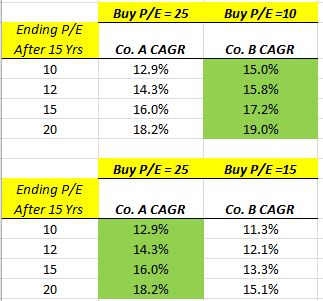
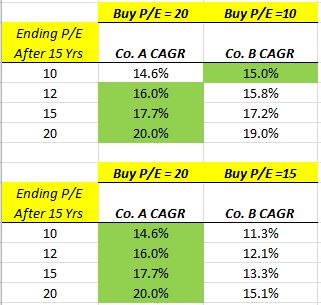
Company A vs. Company B at Various Valuations
The tables compare purchase price P/E’s for Co A and B (the top row) and compares that to various sale P/E’s 15 years later (the left hand column). As you can see, at a certain valuation, Company B is a better investment, but I noticed that you could roughly pay twice the valuation for Company A and still come out ahead over time. And I used 15 years, but the longer you own Company A, the wider the gap gets between Company A’s and Company B’s investment result.
So at a certain gap between the valuation levels, Company B becomes a better investment. But you’d have to pay 2.5 times the valuation of Company B in order to get an inferior result from Company A.
Below, you can see that at twice the valuation (P/E of 20 vs. P/E of 10), it is roughly even. But if you have to pay 15 times earnings for Company B, Company A is a vastly superior investment.
If the ending P/E is the same for both companies (unlikely given the better quality of Company A), Company A still outperforms Company B as an investment, even if Company A’s multiple contracts and Company B’s multiple expands and even though we paid 20 P/E for Company A and just 10 P/E for Company B.
Keep in mind, Company B is still a good company (it’s compounding value at 10%), it’s just not nearly as good as Company A, which will be the superior investment all the way up to twice the price relative to earnings.
“If you’re right about the business, you’ll make a lot of money.” – Warren Buffett, University of Georgia, 2001
Some of you might be rolling your eyes at the simplicity (obviously, high ROIC and the corresponding growth will lead to good investment returns). Other readers are thinking—yeah, owning a great business is great, but the problem is predicting which businesses will be able to sustainably produce these high returns on capital.
It is difficult. But the purpose here is to show the actual math behind the ROIC and the ability of a business to reinvest large portions of earnings and why those two factors are so important to long term owner returns.
This is why Buffett is always talking about great businesses. It’s not just because he wants to sound like a simple, wise, grandfatherly figure--it’s because of the math. If you pick the right business, the multiple you pay for the business is far less significant on your returns than the quality and sustainability of the returns on capital.
Now, I am not recommending paying 25 times earnings for quality businesses. As the skeptics pointed out earlier, it’s far too difficult to predict what the next 15 years will look like.
But that doesn’t mean I want to abandon the effort to locate great businesses. It’s just that ideally, we want to find them cheap enough to secure a large margin of safety in case we are wrong in our assessment of the quality of the business, as this gives us two things:
- A margin of safety if we were wrong about the quality or sustainability of the business’ return on capital
- The benefit of much higher returns if we were in fact right about the business
This is part art, part science, but over time, sticking to businesses that create high returns on the capital it invests will significantly improve the probability of achieving above average investment returns.
In other words, as some investors like to say—and a phrase I often repeat—Heads, we win. Tails, we don’t lose much…
In the next post, we’ll take a look at a real life example (one among many examples), to show that a business that produces consistent returns on capital over time does in fact create real value for its owners.
John Huber is the founder of Saber Capital Management, LLC. Saber is the general partner and manager of an investment fund modeled after the original Buffett partnerships. Saber’s strategy is to make very carefully selected investments in undervalued stocks of great businesses.
John can be reached at [email protected].